Question

The 116-g sample was heated to 94.5°C and placed into a calorimeter containing 72 g of water at 20.0°C. The heat capacity of the calorimeter was 14.7 J/K. The final temperature in the calorimeter was 25.6°C. What is the specific heat capacity (in J/g°C) of the mineral?

Answer 1

0.2212 J/g.k

Step-by-step explanation:

The law of conservation of Energy states that the sum of heat given out by the mineral in the system and the heat absorbed by the water and calorimeter are equal to zero.

This implies that:

`Q_m+Q_w+Q_c=0`

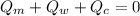
We can as well say that:

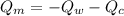`Q_m= -Q_w-Q_c` ----------------equation (1)

Also, heat absorbed by the calorimeter which is denoted by;

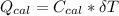`Q_(cal)= C_(cal)* \delta T` ---------------- equation (2)

where;

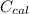`C_(cal)` = heat capacity of the calorimeter

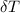`\delta T` = change in temperature

However, the heat released by the mineral and the one absorbed by the water in the system is given as:

Q = mcΔT ------------------ equation (3)

where;

m = mass

c = specific heat capacity

ΔT = change in temperature

If we substitute the two prior equation (i.e equation 2 and 3) into equation 1: we have;

`Q_m=-Q_w-Q_c`

`m_mc_m\delta T_m=-(m_wc_w\delta T_w)-(C_(cal) \delta T_c)`

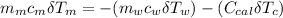
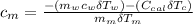`c_m=(-(m_wc_w \delta T_w)-(C_(cal) \delta T_c))/(m_m \delta T_m)` ---------------- equation (4)

Now , let's state our given parameters;

Given that:

mass of water (
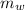`m_w`) = 72g

The specific Heat capacity of water (
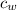`c_w`) = 4.18 J/g.k

change in temperature (
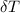`\delta T`) =25.6 °C - 20°C

change in temperature (
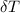`\delta T`) = 5.6 °C

(since we are asked to leave our answer in J/g°C)

Heat capacity of calorimeter
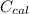`C_(cal)` = 14.7 J/k

change in temperature (
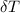`\delta T`) = 5.6 °C

mass of the mineral = 116 g

change in temperature of the mineral (
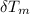`\delta T_m`) = 25.6 °C - 94.5 °C

change in temperature of the mineral (
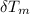`\delta T_m`) = -68.9 °C

specific heat capacity of the mineral

∴ substituting all our values into equation (4); we have:

`c_m=(-(4.18J/g.k*72g*5.6^0C)-(14.7J/k*5.6^0C))/(116g*-68.9^0C)`

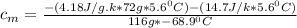
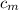`c_m` = 0.221172 J/g°C

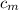`c_m` = 0.2212 J/g°C