Question

The activation energy for the isomerization ol cyclopropane to propene is 274 kJ/mol. By what factor does the rate of this reaction increase as the temperature rises from 255 to 291 oC? Hint: The factor is the ratio of the rates. Since rate is directly proportional to rate constant, the factor is also ratio of rate constants.

Answer 1

53.73 factor

Step-by-step explanation:

Given that:

The activation energy (
`E_a`) = 274 kJ/mol.

T₁ = 255°C = (255+273) = 528 K

T₂ = 291° C = (291 + 273) = 564 K

Rate Constant (R) = 8.314 × 10⁻³ kJ k⁻¹ mol⁻¹

We are tasked to determine the factor at which the rate of the reaction increase as the temperature rises from 255°C to 291° C; In order to do that;

We can apply the use of Arrhenius Equation, which is given by:

㏑
`((K_2)/(K_1))` =
`(E_a)/(R) [(1)/(T_1)-(1)/(T_2)]`

㏑
`((K_2)/(K_1))` =
`(273kJ/mol)/(8.314*10^(-3)kJK^(-1)) [(1)/(528)-(1)/(564)]`

㏑
`((K_2)/(K_1))` =
`(273kJ/mol)/(8.314*10^(-3)kJK^(-1)) [(36k)/((528K)(564K))]`

㏑
`((K_2)/(K_1))` = 3.984

`((K_2)/(K_1))` =
`e^{3.984`

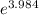
`((K_2)/(K_1))` = 53.73

K₂ = 53.73 K₁

∴ As the temperature rises from 255°C to 291° C, the rate of reaction increases by 53.73 factor