Question

Use the following information to answer Questions 31 and 32. Male players at the high school, college, and professional ranks use regulation basketballs that weigh on average 22.0 ounces with a standard deviation of 1.0 ounce. Assume that the weights of the basketballs are approximately Normally distributed. Roughly what percentage of regulation basketballs weight more than 23.1 ounces?a. 42.396b. 36.496c. 13.696d. 15.196

Answer 1

c. 13.6%

Explanation:

Mean weight (μ)= 22.0 ounces

Standard deviation (σ) = 1.0 ounce

Assuming a normal distribution, for any given weight X, the corresponding z-score is found by the following equation:

`z=(X-\mu)/(\sigma)`

For X = 23.1 ounces:

`z=(23.1-22.0)/(1.0)\\ z=1.1`

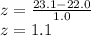
According to a z table, a score of 1.1 corresponds to the 86.43th percentile of a normal distribution. Therefore, the percentage of regulation basketballs that weight more than 23.1 ounces is:

`P(X>23.1) = 1-0.8643=0.1357=13.57\%`

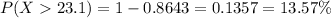
The percentage is 13.6%.