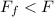1) Available force of friction: 6174 N
2) No
Step-by-step explanation:
1)
The magnitude of the frictional force between the car's tires and the pavement of the road is given by

where
 is the coefficient of friction
is the coefficient of friction
m is the mass of the car
g is the acceleration of gravity
For the car in this problem, we have:
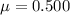 (coefficient of friction)
(coefficient of friction)
m = 1260 kg (mass of the car)

Therefore, the force of friction is

2)
In order to mantain the car in circular motion, the force of friction must be at least equal to the centripetal force.
The centripetal force is given by
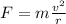
where
m is the mass of the car
v is the tangential speed
r is the radius of the curve
In this problem, we have
m = 1260 kg
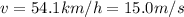 is the tangential speed
is the tangential speed
r = 41.6 m is the radius of the curve
Therefore, the centripetal force is

Therefore, the force of friction is not enough to keep the car in the curve, since