Answer: The
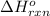 for the reaction is -1.9 kJ.
for the reaction is -1.9 kJ.
Step-by-step explanation:
Hess’s law of constant heat summation states that the amount of heat absorbed or evolved in a given chemical equation remains the same whether the process occurs in one step or several steps.
According to this law, the chemical equation is treated as ordinary algebraic expressions and can be added or subtracted to yield the required equation. This means that the enthalpy change of the overall reaction is equal to the sum of the enthalpy changes of the intermediate reactions.
The given chemical reaction follows:
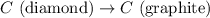
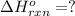
The intermediate balanced chemical reaction are:
(1)
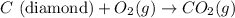
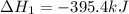
(2)
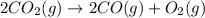
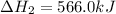 ( × 2)
( × 2)
(3)
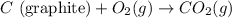
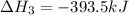
(4)
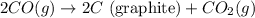
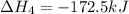 ( × 2)
( × 2)
The expression for enthalpy of the reaction follows:
![\Delta H^o_(rxn)=[1* (\Delta H_1)]+[2* \Delta H_2]+[1* (\Delta H_3)]+[2* \Delta H_4]](https://img.qammunity.org/2021/formulas/chemistry/high-school/uyhx0a05mbtdiz2vfbe8khr8sgs36ocdi1.png)
Putting values in above equation, we get:
![\Delta H^o_(rxn)=[(1* (-395.4))+(2* (566.0))+(1* (-393.5))+(2* (-172.5))]=-1.9kJ](https://img.qammunity.org/2021/formulas/chemistry/high-school/thb94b4gmq2htty52ruhbevawhfhjzijkc.png)
Hence, the
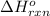 for the reaction is -1.9 kJ.
for the reaction is -1.9 kJ.