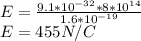Question

An electron is projected horizontally into the uniform electric field directed vertically downward between two parallelplates. The plates are 2.00 cm apart and are of length 4.00 cm. The initial speed of the electron is vi = 8.00 × 106 m/s. As it enters the region between the plates, the electron is midway between the two plates; as it leaves, the electron just misses the upperplate.a)What is the magnitude ofv the electric field?

Answer 1

455N/C

Step-by-step explanation:

from the question, the following data can be derived

Distance between plates=2cm=0.02m

length of plates=4cm=0.04m

initial speed of electron=8*10^6m/s

Note: the speed giving is the speed associated with the horizontal motion since it moves to cover the 4cm distance

we solve the equation component by component.

For the horizontal component, the time it takes to cover distance of 0.04m can be calculated as

`time=(distance )/(velocity) \\t=(0.04)/(8*10^(6))\\ t=5*10^(-9)secs`

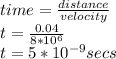
this same time is used to cover the vertical distance which is midway between the plate,

Hence vertical distance covered is 0.02/2=0.01m

The acceleration in the vertical component can be calculated as

`y=ut+1/2at^(2)\\u=0,\\y=0.01m\\a=(2y)/(t^(2))\\ a=(2*0.01)/(5*10^(-9))\\ a=8*10^(14)m/s^(2)`

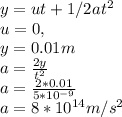
since

F=qE

also F=ma

then

qE=ma

E=(ma)/q

m=mass of electron=9.1*10^-32kq

q=charge of electron=1.6*10^-19c

a=acceleration

if we substitute values

`E=(9.1*10^(-32)*8*10^(14))/(1.6*10^(-19)) \\E=455N/C`