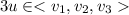Answer:
See proof below
Explanation:
We will use the hint. The statement of the hint holds true, as the linear span of a set of vectors T is equal to the set of linear combinations of vectors in T.
Denote the linear span of vectors with the curly brackets < >, that is,
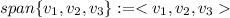
Let
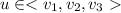 , then u is a linear combination of v1,v2,v3, that is, there exist scalars
, then u is a linear combination of v1,v2,v3, that is, there exist scalars
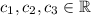 such that
such that
 . Multiply by 3 in both sides to get
. Multiply by 3 in both sides to get
 , with
, with
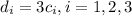
Since
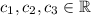 ,
,
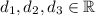 as real numbers are closed under multiplication. Therefore 3u is a linear combination of the vectors
as real numbers are closed under multiplication. Therefore 3u is a linear combination of the vectors
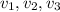 , that is,
, that is,