Answer:
91.02% probability of selling more than 4 properties in one week.
Explanation:
For each property, there are only two possible outcomes. Either it is sold, or it is not. The chance of selling any one property is independent of selling another property. So we use the binomial probability distribution to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.
In this problem we have that:
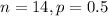
Compute the probability of selling more than 4 properties in one week.
Either you sell 4 or less properties in one week, or you sell more. The sum of the probabilities of these events is decimal 1. So
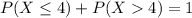
We want to find
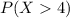 . So
. So
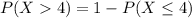
In which
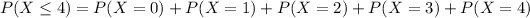
So


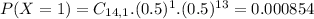
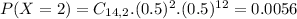
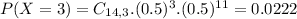
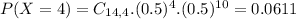
So
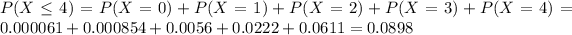
Finally
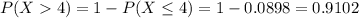
91.02% probability of selling more than 4 properties in one week.