Question

Consider the quadratic function LaTeX: f\left(x\right)=8x^2-7x+6f ( x ) = 8 x 2 − 7 x + 6. What is the constant of the function?

Group of answer choices

6

-7

8

7

Answer 1

Answer:the answer is C

Explanation:

I JUST TOOK THE TEST!

Answer 2

6

Explanation:

Suppose we have a quadratic function f(x) in the following format:

`f(x) = ax^(2) + bx + c, a \\eq 0`

The constant is the value of c.

Also, another way to find the constant is calculating f(0).

In this problem, we have that:

`f(x) = 8x^(2) - 7x + 6`

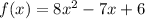
So

`a = 8, b = -7, c = 6`

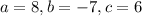
The constant is c = 6.

Also

`f(0) = 8*(0)^(2) - 7*(0) + 6 = 6`

So the correct answer is:

6