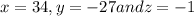Question

Solve the system of equations 2x+2y=6z=8 5x+6y+5z=3 5x+6y+3z=5

Answer 1

`x=34,y=-27andz=-1`

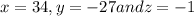
Explanation:

Given:

`2x+2y+6z=8` equation:1

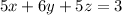`5x+6y+5z=3` equation:2

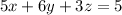`5x+6y+3z=5` equation:3

We have to find the value of x,y and z

Eliminating two variables to find the value of third variable

Subtracting equation:2 from equation:3

`5x+6y+3z-(5x+6y+5z)=5-3`

`5x+6y+3z-5x-6y-5z=2\\-2z=2\\z=-1`

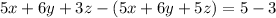
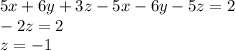
Solving the equation:1 for 'x'

`2x+2y+6z=8`

`2x=8-2y-6z\\2x=8-2y-6(-1)\\2x=8-2y+6\\2x=14-2y\\x=7-y`

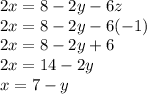
Putting x and z in equation 2

`5(7-y)+6y+5(-1)=3\\35-5y+6y-5=3\\y+30=3\\y=3-30\\y=-27`

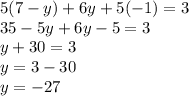
Putting the value of 'y' and 'z' in equation:2 to find the value of 'x'

`5x+6(-27)+5(-1)=3\\5x-162-5=3\\5x-167=3\\5x=3+167\\5x=170\\x=(170)/(5)\\ x=34`

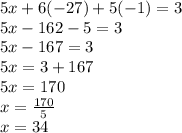
The values of x,y and z are

`x=34,y=-27andz=-1`