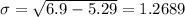Answer:
a) 0.7
b) 2.3
c) 1.2689
Explanation:
a) Find the probability that Albert will tell at least 2 dad jokes in a day.
P(X ≥ 2) = P(X=2)+P(X=3)+P(X=4) = 0.2+0.3+0.2 = 0.7
b) Find the expected number of times Albert will tell a dad joke in a day, E[X].
E[X] = 0*P(X=0)+1*P(X=1)+2*P(X=2)+3*P(X=3)+4*P(X=4) =
= 0.2+2*0.2+3*0.3+4*0.2 = 2.3
c) Find the standard deviation of dad jokes told in a day.
The standard deviation can be defined as
![\sigma=√(E[X^2]-(E[X])^2)](https://img.qammunity.org/2021/formulas/mathematics/college/4qw8a2cqsv93dll7kkalbkxru48obt94va.png)
On one hand we have
![E[X^2]=0^2*0.1+1^2*0.2+2^2*0.2+3^2*0.3+4^2*0.2=6.9](https://img.qammunity.org/2021/formulas/mathematics/college/n292y17hddn9wxt2fhmzup51w5srkmvdev.png)
on the other hand,
![(E[X])^2=(2.3)^2=5.29](https://img.qammunity.org/2021/formulas/mathematics/college/8rip48qcxstjklowgta5pdnfpek94sonpw.png)
So