The concepts used to solve this problem are sound power, area of ear drum, and inverse square law. First find the intensity
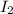 using inverse square law for different distances. Then find the area of the ear drum using the diameter and finally find the energy transferred to each ear drum in one second. Intensity is inversely proportional to the square of the distance from the source:
using inverse square law for different distances. Then find the area of the ear drum using the diameter and finally find the energy transferred to each ear drum in one second. Intensity is inversely proportional to the square of the distance from the source:

Here,
r = Distance from the source,
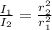

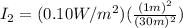

Now the expression for the Area of ear drum is
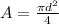
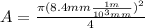

The expression for Sound power is
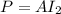
Replacing,

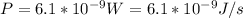
Therefore the energy reached per second is
