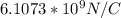Question

A nonconducting wall carries a uniform charge density of 10.81 µC/cm2 . What is the electric field 4.8 cm in front of the wall? The permittivity of a vacuum is 8.8542 × 10−12 C 2 /N · m2 . Answer in units of N/C.

Answer 1

To solve this problem it is necessary to apply the concepts related to electric field due to non conducting wall. This is defined as

`E = (\sigma)/(2\epsilon_0)`

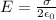
We have that

`\sigma` is the charge per unit area of the wall and
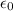`\epsilon_0` the vacuum permittivity

First we need to convert to SI Units,

`\sigma= 10.81 \mu C/cm^2 ((100^2cm^2)/(1m^2))((10^(-6) C)/(1 \mu C))`

`\sigma = 0.1081C/m^3`

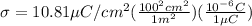
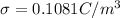
Now replacing we have,

`E = (0.1081C/m^3)/(2(8.85*10^(-12)C^2/Nm^2))`

`E = 6.1073*10^9N/C`

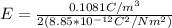
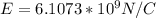
Therefore the electric field is
`6.1073*10^9N/C`