The rate of the cabin cruiser in calm water is 9 mph and the rate of the current 3 mph
Explanation:
Given:
Speed of cabin cruiser travelling with then current = 12 mi in 1 h.
Against the current, it took 2 h to travel the same distance.
To Find:
The rate of the boat in calm water and the rate of the current = ?
Solution:
Let boat speed in calm water be s
Let rate of the current be c
Then
(s-c) = effective speed upstream
and
(s+c) = effective speed downstream
Writing a distance equation for each trip:
distance =
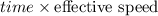
1(s + c) = 12
2(s - c) = 12
On the first equation divide both sides by 1
On the second equation divide both sides by 2
s + c = 12
s - c = 6
Now addition of the above two equations gives
2s = 18
s = 9 mph, boat speed in calm water
Lets substitute s value to find c value
s + c = 12
9 + c = 12
c = 12 - 9
c = 3 mph is the current