Question

Write a quadratic equation and solve it by completing the square. Show your work.

Answer 1

Let the quadratic equation be
`2x^2 +12x+ 6`

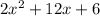
Step 1: Take the coefficients of the
`x^2` term and divide the entire equation

Here the coefficient of
`x^2` is 2

So dividing the entire equation by 2, we get

`x^2 +6x+ 3`

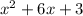
Step 2: Move the constant term to the right side of the equation

`x^2 +6x = - 3`

Step 3: Complete the square on the left side of the equation and balance this by adding the same number to the right side of the equation

`((b)/(2))^2` =
`((6)/(2))^2` =
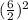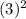`(3)^2` = 9

`x^2 +6x + 9 = - 3 +9`

`(x+3)^2 = 6`

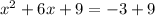
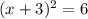
Step 4: Take the square root on both sides of the equation

`(x+3) = √(6)`

`x+3 = \pm 2.45`

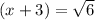
Step 5 Subtract 3 from both sides:

`x+3 - 3 = \pm 2.45 -3`

`x = \pm 2.45 -3`

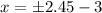
x = 2.45 - 3 or x = -2.45 - 3

x = - 0.55 or x = -5.45