Answer:
38.9 square inches.
Explanation:
We are asked to find the difference in area covered by a single 3 inch windshield wiper operating with a central angle of 138 degrees compared to a pair of 5 inch wipers operating together each having a central angle of 114 degrees.
We will use area of sector formula to solve our given problem as:
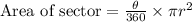 , where, r represents radius and theta represents central angle.
, where, r represents radius and theta represents central angle.
Let us find area of sector with central angle 140 degree and radius 3 inch.
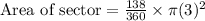

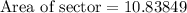
Now, we will find area of sector with central angle 114 degree and radius 5 inch and multiply by 2 as:
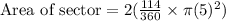
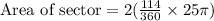
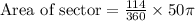

Let us find difference of area as shown below:
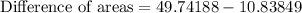
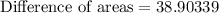
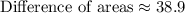
Therefore, the difference in area covered is approximately 38.9 square inches.