Answer:
Common difference(d) is:

d is constant till
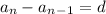
Explanation:
An arithmetic sequence or arithmetic progression is the sequence, whose difference of consecutive terms called common difference is constant throughout the sequence.
Considering, the sequence
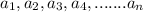

d= common difference.
we can find any
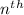 term in the sequence by using the formula
term in the sequence by using the formula

where
 = any
= any
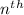 term
term
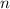 =Number of terms
=Number of terms
 =common difference
=common difference
 = first term
= first term
Also sum of n terms of A.P can be find by the formula:
![S_n=(n)/(2) [2a+(n-1)d]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/agc7i8topn33t5o2cxb1z6ccneavuo4y66.png)