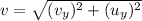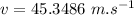Question

A ball is thrown horizontally from the top of a 50.5-m building and lands 104.8 m from the base of the building. Ignore air resistance. (Assume the ball is thrown in the x direction and upward to be in the y direction.)a. What must have been the initial horizontal component of the velocity? b. What is the vertical component of the velocity just before the ball hits the ground? c. What is the velocity (including both the horizontal and vertical components) of the ball just before it hits the ground?

Answer 1

a.
`u_x=32.6448\ m.s^(-1)`

b. Zero

c.
`v=45.3486\ m.s^(-1)`

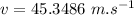
Step-by-step explanation:

Given:

• height of the building form where the ball is thrown horizontally,
  `h=50.5\ m`

• horizontal distance of the ball form the base of the building,
  `s=104.8\ m`

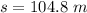
b.

Since the ball is projected horizontally it will have only the initial component of the velocity and zero vertical component of the velocity initially.

So, the initial vertical component of the velocity,
`u_y=0`

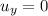
a.

Now we find the time taken by the ball to reach the ground:

`h=u_y.t_d+(1)/(2) * g.t_d^2`

`50.5=0+0.5* 9.81* t_d^2`

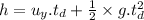
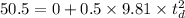
`t_d=3.21\ s` is the time of total motion of the ball.

• Since the horizontal component of the velocity is un-accelerated:

`u_x=v_x`

where:

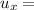`u_x=` initial velocity in the horizontal direction

`v_x=` final velocity in the horizontal direction

`s=u_x* t_d`

`104.8=u_x* 3.21`

`u_x=32.6448\ m.s^(-1)`

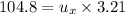
c.

Now the final vertical velocity:

`v_y^2=u_y^2+2g.h`

`v_y^2=0^2+2* 9.81* 50.5`

`v_y=31.4771\ m.s^(-1)`

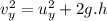
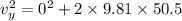
Hence the resultant velocity just before the ball lands:

`v=√((v_y)^2+(u_y)^2)`

`v=√((31.4771)^2+(32.6448)^2)`

`v=45.3486\ m.s^(-1)`