Question

Consider the following intermediate chemical equations.

What is the enthalpy of the overall chemical reaction?
-205.7 kJ
-113.4 kJ
-14.3 kJ
78.0 kJ

Answer 1

The first choice,
`-205.7\; \rm kJ`.

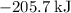Step-by-step explanation:

Let the three reactions, where the enthalpy change were known, be called (1), (2), and (3).

The goal is to find the enthalpy change of the fourth equation. Assume that this equation can be written as
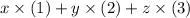`x * (1) + y * (2) + z * (3)` for some
`x`,
`y`, and
`z` (might not be whole numbers or take positive values.) Then, by Hess's Law, the enthalpy change of that reaction would be
`x \cdot \Delta H_1 + y \cdot \Delta H_2 + z \cdot \Delta H_3`.

To find these
`x`,
`y`, and
`z`, consider: what combination of reaction (1), (2), and (3) would give the fourth reaction?

Imagine that the coefficients are positive for all the reactants, and negative for all the products.

For example: in (1),
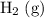`\rm H_2\; (g)` has a coefficient of
`2`. However, since it is on the the product side of (1), its value should be
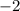`-2`. Also, in (3)

Since there is no
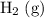`\rm H_2\; (g)` in the desired equation, the value of
`x`,
`y`, and
`z` should ensure that
`-2x + z = 0`.

Another example:
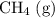`\rm CH_4\; (g)` is on the reactant side of the first reaction. Its coefficient in the equation is
`1`, so that corresponds to
`+1`. Since
`\rm CH_4` is neither in (2) nor in (3), the value of

In the desired equation,
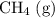`\rm CH_4\; (g)` is on the reactant side with a coefficient of
`1`. As a result, the value of
`x`,
`y`, and
`z` should ensure that
`x = 1`.

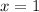One such equation can be found for each species in the reactions.

`\displaystyle \begin{cases}x = 1 & \left(\text{For $\mathrm{CH_4\; (g)}$}\right) \\ -x - y= 0 & \left(\text{For $\mathrm{C\; (s)}$}\right) \\ -2\, x + z = 0 & \left(\text{For $\mathrm{H_2\; (g)}$}\right) \\ y = -1 & \left(\text{For $\mathrm{CCl_4\; (g)}$}\right) \\ -2\, y + z= 4 & \left(\text{For $\mathrm{Cl_2\; (g)}$}\right) \\ -2\, z = -4 & \left(\text{For $\mathrm{HCl\; (g)}$}\right)\end{cases}`.

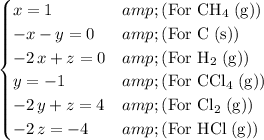Solve this system of equations for
`x`,
`y`, and
`z` (this approach works only if at least one solution exists.) In this case,

`\displaystyle \begin{cases}x = 1 \\ y = -1 \\ z = 2\end{cases}`.

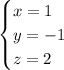Calculate the enthalpy change of the desired reaction:

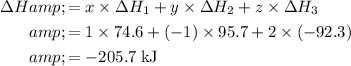`\begin{aligned}\Delta H &= x* \Delta H_1 + y * \Delta H_2 + z * \Delta H_3 \\ &= 1 * 74.6 + (-1) * 95.7 + 2 * (-92.3) \\ &= -205.7\; \rm kJ\end{aligned}`.