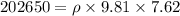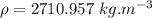Question

A cylindrical tank filled to a height of 25 feet with tribromoethylene has been pressurized to 3 atmospheres (Psurface= 3 atmospheres). The total pressure at the bottom of the tank is 5 atmospheres. Determine the density of tribromoethylene in units of kilogrms per cubic meter.

Answer 1

`\rho=2710.957\ kg.m^(-3)`

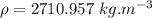
Step-by-step explanation:

Given:

height of the given liquid in the tank,
`h=25\ ft=7.62\ m`

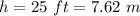
pressure at the surface of the liquid,
`P_(surf)=3\ atm`

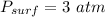
pressure at the bottom of the liquid,
`P_(botm)=5\ atm`

So the pressure due to height of the liquid column:

`\Delta P=P_(botm)-P_(surf)`

`\Delta P=5-3`

`\Delta P= 2\ atm=202650\ Pa`

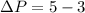
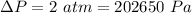
Now as we know that the pressure due to the height of liquid column is given as:

`\Delta P=\rho.g.h`

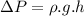
where:

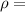`\rho=` density of the liquid

`g=` acceleration due to gravity

`202650=\rho* 9.81* 7.62`

`\rho=2710.957\ kg.m^(-3)`