The given question is incomplete. The complete question is as follows.
A 56-kg hiker is climbing the 828-m-tall Burj Khalifa in Dubai. If the efficiency of converting the energy content of the bars into the work of climbing is 25%, the remaining 75% of the energy released through metabolism is heat released to her body. She eats two energy bars, one of which produces 1.10×103kJ of energy upon metabolizing. Assume that the heat capacity of her body is equal to that for water (75.3 Jmol−1⋅K−1). Calculate the increase in her temperature at the top of the structure.
Calculate the temperature at the top of the structure. Assume her intitial temperature to be
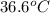 .
.
Step-by-step explanation:
Energy present in total of two bars is as follows.
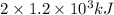
 kJ
kJ
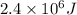 (as 1 kJ = 1000 J)
(as 1 kJ = 1000 J)
As 75% of energy releases out. Let us assume that energy releases out of its body be E. Then, energy will be calculated as follows.
E =

=
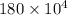 J
J
Given data is as follows.
mass (m) = 56 kg
= 56000 g (1 kg = 1000 g)
Specific heat = 4.18
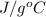
As heat is releasing which means that value of E will be negative.
-E =
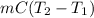
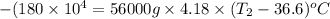
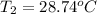
Her temperature at the top of the structure is
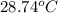 .
.
Now, change in temperature is calculated as follows.

=
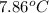
Thus, we can conclude that increase in her temperature at the top of the structure is
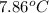 .
.