Question

A stone thrown horizontally from a height of 6.22 m hits the ground at a distance of 11.90 m. Calculate the initial speed of the stone. Neglect air resistance.

Answer 1

10.625m/s

Step-by-step explanation:

Using one of the equations of motion as follows;

h = u t +
`(1)/(2)` a t² -------------------(i)

Where;

h = horizontal/vertical distance covered

u = initial velocity of the object(stone)

t = time taken to cover the distance

a = acceleration of the object.

Taking the horizontal motion of the stone;

h = horizontal distance of the stone = 11.90m

a = acceleration = 0 (the horizontal motion is at constant velocity, therefore, acceleration is 0).

Substitute these values into equation(i) to give;

11.9 = u t + 0

11.9 = ut ------------------------------(ii)

Taking the vertical motion of the stone,

h = height from which the stone was thrown = 6.22m

a = acceleration due to gravity = g = +10m/s² (since the stone moves downwards in the direction of gravity)

u = 0 (since the ball was thrown horizontally, the vertical component of the velocity, u, is 0)

Substitute these values into equation (i) to give;

6.22 = 0 x t +
`(1)/(2)` x 10 x t²

6.22 = 5 x t² --------------------(iii)

Solve for t;

6.22 = 5t²

5t² = 6.22

t² = 6.22 / 5

t² = 1.244

t =
`√(1.244)`

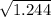
t = 1.12s

Since it takes the same time for the stone to hit the ground as it will take it to move the horizontal distance of 11.9, therefore we can substitute the time t = 1.12s into equation (ii) as follows;

=> 11.9 = ut

=> 11.9 = u (1.12)

=> u = 11.9 / 1.12

=> u = 10.625m/s

Therefore, the initial speed of the stone is 10.625m/s

Answer 2

v = 10.56 m/s

The initial speed of the stone is 10.56 m/s

Step-by-step explanation:

We need to determine the time of flight;

The vertical height covered is h = 6.22 m

initial vertical speed u = 0

Using the equation of motion;

s = ut + 0.5at^2 ......1

Where;

height s = h

u = 0

a = g acceleration due to gravity = 9.8m/s^2

The equation 1 becomes;

h = 0.5gt^2

making t the subject of formula:

t = √(2h/g)

Substituting the values:

t = √(2×6.22/9.8)

t = 1.127s

The horizontal distance covered can be written as;

R = vt ......2

R = horizontal distance = 11.90m

v = initial horizontal speed

t = time of flight = 1.127s

From equation 2, making v the subject of formula;

v = R/t

v = 11.90m/1.127s

v = 10.56 m/s

The initial speed of the stone is 10.56 m/s