The missing figure is attached down
Answer:
The most specific name for quadrilateral ABCD is parallelogram
Explanation:
The quadrilateral is a parallelogram if
- Its two diagonals bisect each other
- Its two diagonals not equal in length
- Its two diagonals not perpendicular
From the attached figure
∵ The diagonals of the quadrilateral are AC and BD
∵ A = (-2 , 3) , C = (0 , -3)
- Find the slope of AC and its length using the rule of the slope
and the rule of the distance
∵
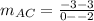
∴
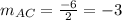
∵
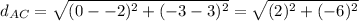
∴
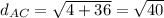
- Find the mid-point of AC
∵

∴
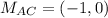
∵ B = (2 , 2) , C = (-4 , -2)
- Find the slope of AC and its length using the rule of the slope
and the rule of the distance
∵
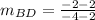
∴
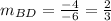
∵
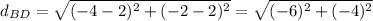
∴
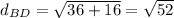
- Find the mid-point of AC
∵
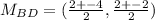
∴
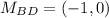
∵
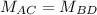 ⇒ diagonals bisect each other
⇒ diagonals bisect each other
∵
 ≠
≠
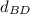 ⇒ diagonals not equal in length
⇒ diagonals not equal in length
∵ The product of their slopes = -3 ×
 = -2
= -2
∵ The product of the slopes of the perpendicular lines is -1
∴ AC and BD are not perpendicular
∴ ABCD is a parallelogram
The most specific name for quadrilateral ABCD is parallelogram