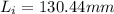Question

A cylindrical specimen of a hypothetical metal alloy is stressed in compression. If its original and final diameters are 17.847 and 17.869 mm, respectively, and its final length is 72.0 mm, calculate its original length if the deformation is totally elastic. The elastic and shear moduli for this alloy are 109 GPa and 40.0 GPa, respectively.

Answer 1

`L_i  = 130.44 mm`

Step-by-step explanation:

Given data:

original diameter = 17.847mm

final diameter = 17.869 mm

final length = 75mm

elastic modulus = 109 GPa

shear modulus = 40 GPa

we know

`E = 2G( 1 + \\u)`

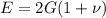
solving for Poisson's ratio

`\\u = \frac{E}[2G} - 1`

`\\u = (109)/(2* 40) -1 = 0.362`

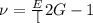
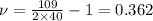
we know that Poisson ration is given as

`\\u = \rac{\frac{d_f -d_i}[d_i}}{\frac{L_f -L_i}[L_i}}`

`0.362 = - ((17.869 - 17.847)/(17.847))/((75 - L_i)/(L-i))`

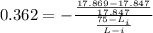
solving initial length

`L_i  = 130.44 mm`