Answer:
0.95 A
Step-by-step explanation:
P = Power of bulb = 4 W
V = Voltage = 12 V
Power is given by
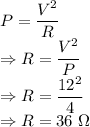
The connections are in Parallel
Equivalent resistance of these two bulb
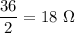
Current from the source
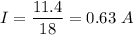
The voltage drop is

Voltage drop is given by
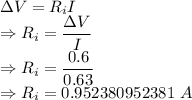
The internal resistance is 0.95 A