Answer:
A) At t2 = 22.7 s, the x-component of the velocity is 7.3 m/s and the y-component is 0.7 m/s.
B) The magnitude of the dog's velocity is 7.3 m/s.
C) The direction of the velocity vector is 5.5° from the x-axis to the y-axis.
Step-by-step explanation:
Hi there!
The components of the velocity vector can be calculated using this equations:
vx = v0x + ax · t
vy = v0y + ay · t
Where:
vx = x-component of the velocity
v0x = initial x-component of the velocity.
ax = x-component of the acceleration.
vy = y-component of the velocity.
v0y = initial y-component of the velocity.
ay = y-component of the acceleration.
t = time
A) Let's calculate the velocity using the equations. The initial velocity vector is v0 = (2.8, -2.3) m/s. The elapsed time is (22.7 s - 10.3 s) 12.4 s.
To calculate the components of the acceleration vector, we use trigonometry:
cos θ = ax/a (where "a" is the magnitude of the acceleration)
ax = a · cos θ
ax = 0.43 m/s² · cos(33.5°) = 0.36 m/s²
ay = a · sin θ
ay = 0.43 m/s² · sin(33.5°) = 0.24 m/s²
Now, we can calculate the velocity:
vx = v0x + ax · t
vx = 2.8 m/s + 0.36 m/s² · 12.4 s
vx = 7.3 m/s
vy = v0y + ay · t
vy = -2.3 m/s + 0.24 m/s² · 12.4 s
vy = 0.7 m/s
At t2 = 22.7 s, the x-component of the velocity is 7.3 m/s and the y-component is 0.7 m/s.
b) The magnitude of the velocity is calculated as follows:
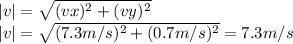
The magnitude of the dog's velocity is 7.3 m/s
c) Now that we have the magnitude of the velocity, we can calculate its direction:
sin θ = vy / v
sin θ = 0.7 m/s / 7.3 m/s
θ = 5.5°
The direction of the velocity vector is 5.5° from the x-axis to the y-axis.