- The magnitude of r is 7.32 m.
- If the centripetal acceleration is directed due East, the direction of r is West.
- If the centripetal acceleration is directed due South, the direction of r is North.
Step-by-step explanation:
Given, constant speed v = 3.36 m / s, centripetal acceleration a = 1.86 m/s^2.
- a) The magnitude of position vector r is relative to the rotational axis is the radius of circular path.
|a| =
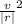
|r| =
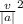 = (3.66)^2 / 1.86
= (3.66)^2 / 1.86
|r| = 7.32 m.
The centripetal acceleration and radius vector are in opposite directions and the direction of centripetal acceleration is always inwards along radius vector.
- b) If the centripetal acceleration is directed due East, the direction of r is West.
- If the centripetal acceleration is directed due South, the direction of r is North.