Question

Lines AC←→ and DB←→ intersect at point W. Also, m∠DWC=138° .

Enter the angle measure for the angle shown.

Answer 1

ADW = 42

AWB = 138

BWC = 42 It worked for me, hope it works for you.

Explanation:

Have FAITH!

Answer 2

m∠DWC=138°, ∠AWB = 138°, ∠AWD = 42°, ∠BWC = 42°

Solution:

Line
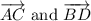`\overrightarrow{A C} \text { and } \overrightarrow{B D}` intersect at a point W.

Given
`m \angle D W C=138^(\circ)`.

Vertical angle theorem:

If two lines intersect at a point then vertically opposite angles are congruent.

To find the measure of all the angles:

∠AWB and ∠DWC are vertically opposite angles.

Therefore, ∠AWB = ∠DWC

⇒ ∠AWB = 138°

Sum of all the angles in a straight line = 180°

⇒ ∠AWD + ∠DWC = 180°

⇒ ∠AWD + 138° = 180°

⇒ ∠AWD = 180° – 138°

⇒ ∠AWD = 42°

Since ∠AWD and ∠BWC are vertically opposite angles.

Therefore, ∠AWD = ∠BWC

⇒ ∠BWC = 42°

Hence the measure of the angles are

m∠DWC=138°, ∠AWB = 138°, ∠AWD = 42°, ∠BWC = 42°.