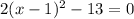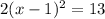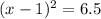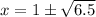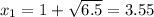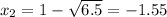Question

Find the x-intercepts of the parabola with

vertex (1,-13) and y-intercept (0.-11).
Write your answer in this form: (X1.),(X2,92).
If necessary, round to the nearest hundredth.
Enter the correct answer.​

Answer 1

`x_1=3.55`

`x_2=-1.55`

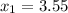
Explanation:

we know that

If the vertex is (1,-13) and the y-intercept is (0.-11) (y-intercept above the vertex), we have a vertical parabola open upward

The equation of a vertical parabola written in vertex form is equal to

`y=a(x-h)^2+k`

where

a is a coefficient

(h,k) is the vertex

substitute the given value of the vertex

`y=a(x-1)^2-13`

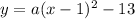
Find the value of a

Remember that we have the y-intercept

For x=0, y=-11

substitute

`-11=a(0-1)^2-13`

`a=13-11\\a=2`

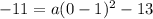
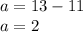
so

`y=2(x-1)^2-13`

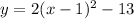
Find the x-intercepts

For y=0

`2(x-1)^2-13=0`

`2(x-1)^2=13`

`(x-1)^2=6.5`

`x-1=\pm√(6.5)`

`x=1\pm√(6.5)`

`x_1=1+√(6.5)=3.55`

`x_2=1-√(6.5)=-1.55`