Answer:
a)
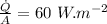
b)
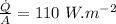
c) The wall may not be under steady because the two surfaces of the wall are exposed to the air at different temperatures and they have different convective coefficient.
Step-by-step explanation:
Given:
- temperature of the inner surface of the wall,
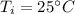
- temperature of the outer surface of the wall,
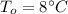
- temperature of the air outside,
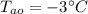
- temperature of the air inside,

- coefficient of heat convection on outside,
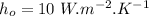
- coefficient of heat convection on inside,
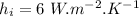
a)
The heat flux between the interior air and the wall:
The convective heat transfer rate is given as,
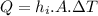
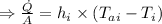
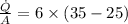
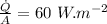
b)
The heat flux between the exterior air and the wall:
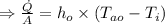
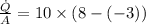
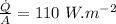
c)
The wall may not be under steady because the two surfaces of the wall are exposed to the air at different temperatures and they have different convective coefficient.