Question

Of all 40-year-old women, 1% have breast cancer. If a woman has breast cancer, a mammogram will give a positive indication for cancer 90% of the time. If a woman does not have breast cancer, a mammogram will give a positive indication for cancer 9% of the time. If a 40-year-old mammogram gives a positive indication for cancer, what is the probability that she has cancer?

Answer 1

9.17%

Explanation:

The probability of a positive indication by a mammogram is given by the probability of positive indication when a woman has cancer, added to the probability of positive indication when a woman does not have cancer.

`P(+) = P(C)*P(+_C)+(1-P(C))*P(+_n)\\P(+) = 0.01*0.9+(1-0.01)*0.09\\P(+) = 0.0981`

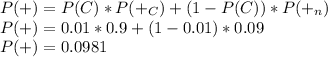
Given that the indication is positive, the probability that a woman actually has cancer is:

`P(C|+) = (P(C)*P(+_C))/(P(+))\\ P(C|+) =(0.01*0.9)/(0.0981)\\P(C|+)= 0.0917 = 9.17\%`

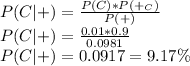
The probability that the randomly selected woman has cancer is 9.17%