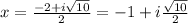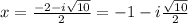Question

What are the roots of 2x^2+4x+7? Use the quadratic formula. Show your work

Answer 1

The roots are

`x=-1+i(√(10))/(2)`

`x=-1-i(√(10))/(2)`

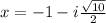
Explanation:

we have

`2x^2+4x+7`

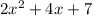
To find the roots equate the equation to zero

so

`2x^2+4x+7=0`

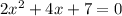
we know that

The formula to solve a quadratic equation of the form

`ax^(2) +bx+c=0`

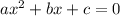
is equal to

`x=\frac{-b\pm\sqrt{b^(2)-4ac}} {2a}`

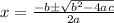
in this problem we have

`2x^2+4x+7=0`

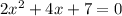
so

`a=2\\b=4\\c=7`

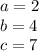
substitute in the formula

`x=\frac{-4\pm\sqrt{4^(2)-4(2)(7)}} {2(2)}`

`x=\frac{-4\pm√(-40)} {4}`

Remember that

`i=√(-1)`

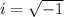
so

`x=\frac{-4\pmi√(40)} {4}`

`x=\frac{-4\pm2i√(10)} {4}`

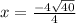
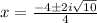
simplify

`x=\frac{-2\pm i√(10)} {2}`

therefore

The roots are

`x=\frac{-2+i√(10)} {2}=-1+i(√(10))/(2)`

`x=\frac{-2-i√(10)} {2}=-1-i(√(10))/(2)`