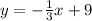Question

Line K has a slope of 3. Line J is perpendicular to like K and passes through the point (3,8). Type the equation of the line in y=Mx+b

Answer 1

I'm assuming you are finding the equation of the line of Line J

Slope-intercept form: y = mx + b

[m is the slope, b is the y-intercept or the y value when x = 0 --> (0, y) or the point where the line crosses through the y-axis]

For lines to be perpendicular, the slopes have to be negative reciprocals of each other. (basically changing the sign (+/-) and flipping the fraction/switching the numerator and the denominator)

For example:

slope = 2 or
`(2)/(1)`

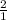
Perpendicular line's slope =
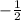`-(1)/(2)` [changed sign from + to -, and flipped the fraction]

slope =
`-(2)/(5)`

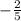
Perpendicular line's slope =
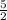`(5)/(2)` [changed sign from - to +, and flipped the fraction]

Since you know the slope is 3, the perpendicular line's slope is
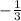`-(1)/(3)`. Plug this into the equation

y = mx + b

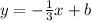`y=-(1)/(3) x+b` To find b, plug in the point (3, 8)

`8=-(1)/(3)(3)+b`

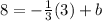
8 = -1 + b Add 1 on both sides to get "b" by itself

8 + 1 = -1 + 1 + b

9 = b

`y=-(1)/(3) x+9`