Question

Imagine that you attempted to recreate Mendel's work with garden peas. You began by crossing true breeding violet-flowered, tall plants with white-flowered dwarf plants.

After self-crossing the F1 generation, you obtain the following phenotypes in the F2generation:

80 tall, violet flowers
36 tall, white flowers
39 dwarf, violet flowers
5 dwarf, white flowers

Part A

How many tall plants with violet flowers were expected?

Express your answer as a whole number.

Part B

How many tall plants with white flowers were expected?

Express your answer as a whole number.

Part C

How many dwarf plants with violet flowers were expected?

Express your answer as a whole number.

Part D

How many dwarf plants with white flowers were expected?

Express your answer as a whole number.

Part E

Use a chi-square analysis to test the hypothesis that the F2 data for stem length (tall:dwarf) is consistent with Mendel's law of segregation.

Calculate the chi square value.

Express your answer to two decimal points (example: 100.00).

Part F

Can the deviation of observed values from expected values be attributed to random chance?

yes or NO

Use the probability chart to answer this question.

Use the probability chart to answer this question.

yes
no
Part G

Use a chi-square analysis to test the hypothesis that the F2 data for stem length (tall:dwarf) and flower color (violet:white) is consistent with Mendel's law of independent assortment.

Calculate the chi square value.

Express your answer to two decimal points (example: 100.00).

Part H

Can the deviation of observed values from expected values be attributed to random chance?

Use the probability chart to answer this question.

Use the probability chart to answer this question.

no
yes

Answer 1

A. 90

B. 30

C. 30

D. 10

E. 0.53

F. Yes

G. 7.51

H. Yes

Step-by-step explanation:

Part A

There are a total of 160 plants (80 + 36 + 39 + 5 = 160).

In Mendel's experiment, an F1 generation of a dihybrid cross between two true breeding plants gives 100% heterozygous plants. We self-cross them to create the F2, in which we expect a ratio of 9:3:3:1. The dominant parental phenotype would be 9, the two recombinant phenotypes would both be 3, and the recessive parental phenotype would be 1.

Therefore, we calculate the total number of plants divided by the total number of potential outcomes from the cross (16), multiplied by 9, which is the expected number of tall violet plants

160 / 16 = 10

10 x 9 = 90

Part B

We carry out this calculation as above, within Mendel's law of 9:3:3:1, tall plants with white flowers represent the recombinant phenotype, meaning we divide the total number of flowers by the total number of potential outcomes from the cross (160/16 = 10). We then multiply this by the expected ratio, which is 3

10 x 3 = 30

Part C

This phenotype is also a recombinant phenotype, meaning the expected ratios are as in part B : dwarf plants with violet flowers represent the recombinant phenotype, meaning we divide the total number of flowers by the total number of potential outcomes from the cross (160/16 = 10). We then multiply this by the expected ratio, which is 3

10 x 3 = 30

Part D

Dwarf plants with white flowers are the recessive parental phenotype, within the ratio of 9:3:3:1, they represent 1. Again, we divide the total number of flowers by the total number of potential outcomes from the cross (160/16 = 10). We then multiply this by the expected ratio, which is 1

10 x 1 = 10

Part E

Mendel's law of segregation states that alleles segregate randomly into gametes. To assess whether the law of segregation is being adhered to here, in terms of stem length, we perform a chi squared test, which has the formula

Sum of =
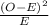`((O-E)^(2))/(E)` for all classes

Where O is the observed value and E is the expected value. The easiest way to do this is to construct a simple table:

The observed number of tall flowers is 80 + 36 = 116, the expected number is 90 + 30 = 120. The observed number of dwarf flowers is 39 + 5 = 44. The expected number of dwarf flowers is 30 + 10 = 40

O E (O-E)² (O-E)²/ E

tall flowers . 116 120 16 0.13

dwarf flowers 44 40 16 0.4

TOTAL = 0.53

Part F

To see whether the deviation of the observed values from the expected values can be attributed to random chance, we first have to know the degrees of freedom.

The degrees of freedom are the number of tested outcomes - 1. In this case there are 2 phenotypes, so 1 degree of freedom.

We then consult a table showing the critical values of the chi squared distribution, to see the maximum chi squared value for our degrees of freedom, within our threshold for statistical significance. A table is attached. The table tells you the probability that the chi squared value was exceeded by random chance.

Our value of 0.53 corresponds to a p value of more than 0.5. This means that, if the law of segregation is true, then deviations from expected values this large are expected approximately 50 percent of the time. our level for significance is 0,05 (5%), so the null hypothesis cannot be rejected and the law of segregation applies.

Part G

Mendel's law of independent assortment states that any combination of alleles from the parents can be inherited in the offspring. I.e. the offspring have just as much chance of inheriting both tall white alleles as both dwarf violet alleles.

Sum of =
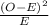`((O-E)^(2))/(E)` for all classes

O E (O-E)² (O-E)²/ E

tall, violet flowers 80 90 100 1.11

tall, white flowers 36 30 36 1.2

dwarf, violet flowers 39 30 81 2.7

dwarf, white flowers 5 10 25 2.5

TOTAL = 7.51

Part H

The degrees of freedom are the number of tested outcomes - 1. In this case there are 4 phenotypes, so 3 degrees of freedom.

Our threshold for significance (p value) is 0.05 unless told otherwise. Our chi squared value corresponds to a p value between 0.1 and 0.05, meaning that deviations from expected values this large are expected approximately 5-10% of the time. This is within our significant threshold, of 0.05 (5%) so our deviations can be attributed to random chance.