Question

Random samples of size 36 are taken from an infinite population whose mean and standard deviation are 20 and 15, respectively. The distribution of the population is unknown. The mean and the standard error of the mean are

Select one:

a. 36 and 15

b. 20 and 15

c. 20 and 2.5

d. 20 and 0.417

Answer 1

The correct option is (c) 20 and 2.5.

Explanation:

The sample size is, n = 36.

The sample mean is,
`\mu=20`

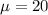
The sample standard deviation is,
`\sigma_(x)=15`

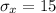
According to the Central limit theorem, if a random sample of size ≥ 30 is selected from an unknown population, then the sampling distribution of the sample means (
`\bar x`) follows a Normal distribution with mean
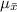`\mu_(\bar x)` and standard deviation
`\sigma_(\bar x)`.

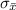The mean is,
`\mu_(\bar x)=\mu =20`

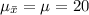
The standard deviation of the sampling distribution of the sample means is known as standard error.

The standard error is:

`\sigma_(\bar x)=(\sigma_(x))/(√(n) ) =(15)/(√(36) )= 2.5`

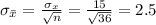
Thus, the mean is 20 and the standard error is 2.5.