Question

In one town, the number of pounds of sugar consumed per person per year has a mean of 8 pounds and a standard deviation 3 of 20 pounds Tyler consumed 13 pounds of sugar last year How many standard deviations from the mean is that? Round your4 answer to two decimal places

A. 250 standard deviations above the mean
B. 2.50 standard deviations below the mean
c. 167 standard deviations above the mean
D. 167 standard deviations below the mean

Answer 1

c. 1.67 standard deviations above the mean

Explanation:

The z-score measures how many standard deviations a score X is from the mean. It is given by the following formula:

`Z = (X - \mu)/(\sigma)`

In which
`\mu` is the mean and
`\sigma` is the standard deviation.

A positive z-score means that X is above the mean, and a negative Z-score means that X i below the mean.

In this problem, we have that:

`\mu = 8, \sigma = 3`

Tyler consumed 13 pounds of sugar last year How many standard deviations from the mean is that?

This is Z when X = 13. So

`Z = (X - \mu)/(\sigma)`

`Z = (13 - 8)/(3)`

`Z = 1.67`

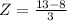
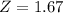
So this is 1.67 standard deviations above the mean.