Question

Two teaching methods and their effects on science test scores are being reviewed. A random sample of 11 students, taught in traditional lab sessions, had a mean test score of 76.1 with a standard deviation of 4.4. A random sample of 13 students, taught using interactive simulation software, had a mean test score of 87.1 with a standard deviation of 5.8. Do these results support the claim that the mean science test score is lower for students taught in traditional lab sessions than it is for students taught using interactive simulation software? Let µ1be the mean test score for the students taught in traditional lab sessions and µ2 be the mean test score for students taught using interactive simulation software. Use a significance level of α = 0.05 or the test. Assume that the population variances are equal and that the two populations are normally distributed.Step 1 of 4: State the null and alternative hypotheses for the test.Step 2 of 4: Compute the value of the t test statistic. Round your answer to three decimal places.Step 3 of 4: Determine the decision rule for rejecting the null hypothesis H0. Round your answer to three decimal places.Step 4 of 4: State the test's conclusion.

Answer 1

Null Hypothesis is rejected that concluded that mean science test score is lower for students taught in traditional lab sessions than it is for students taught using interactive simulation software.

Explanation:

We are given that two teaching methods and their effects on science test scores are being reviewed.

Also,
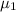`\mu_1` = mean test score for the students taught in traditional lab sessions.

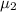`\mu_2` = mean test score for students taught using interactive simulation

software.

Null Hypothesis,
`H_0` :
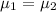`\mu_1 = \mu_2` {Both traditional lab sessions and interactive

simulation software has same mean test score}

Alternate Hypothesis,
`H_1` :
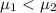`\mu_1 < \mu_2` {Students taught in traditional lab sessions has lower mean science test score than students taught using interactive simulation software}

The test statistics we use here will be :

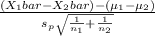`\frac{(X_1bar - X_2bar) - (\mu_1 - \mu_2)}{s_p\sqrt{(1)/(n_1)+ (1)/(n_2) } }` follows
`t_n__1+n_2 -2`

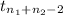
where,
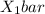`X_1bar` = 76.1 and
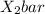`X_2bar` = 87.1

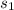`s_1` = 4.4 and
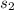`s_2` = 5.8

`n_1` = 46 and
`n_2` = 46

`s_p = \sqrt{((n_1-1)s_1^(2) + (n_2-1)s_2^(2) )/(n_1 +n_2 -2) }` =
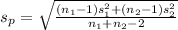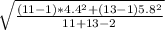`\sqrt{((11-1)*4.4^(2) + (13-1)5.8^(2) )/(11+13 -2) }` = 5.210

Here, we use t test statistics because we know nothing about population standard deviations.

Test statistics =
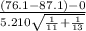`\frac{(76.1 - 87.1) - 0}{5.210\sqrt{(1)/(11)+ (1)/(13) } }` follows
`t_2_2`

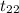
= -5.154

At 0.05 or 5% level of significance t table gives a critical value of -1.717 at 22 degree of freedom. Since our test statistics is less than the critical table value of t as -5.154 < -1.717 so we have sufficient evidence to reject null hypothesis as it will fall in the rejection region.

Therefore, we conclude that mean science test score is lower for students taught in traditional lab sessions than it is for students taught using interactive simulation software.