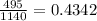Question

Three distinct integers are chosen at random from the first 20 positive integers. Compute the probability that: (a) their sum is even; (b) their product is even.

Answer 1

Explanation:

There are first 20 positive integers 1,2...20. Three distinct integers are chosen at random.

Total no of ways of drawing 3 integers =
`20C3 = 1140`

To Compute the probability that: (a) their sum is even

a) Sum can be even if either all 3 are even or 1 is even and 2 are odd.

There are in total 10 odd and 10 even.

Ways of sum even =
`10C3 + 10C2 (10C1)\\= 120+45(10)\\= 570`

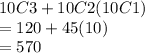
Prob =
`(570)/(1140) =0.50`

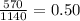
b) their product is even

Here any one should be even or both

SO no of ways are

=
`10C2 +10C2(10C1)\\= 45 +450\\=495`

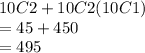
Prob =
`(495)/(1140) =0.4342`