Question

Given the value of cos 20° ≈ 0.9397, enter the sine of a complementary angle. Use an expression relating trigonometric ratios of complementary angles.

Answer 1

cos(20) = sin(70)

note how 20+70 = 90

The general rule is that if x+y = 90, then cos(x) = sin(y). The rule works in reverse as well. Another way to phrase the rule is cos(x) = sin(90-x).

So,

`\cos 20^(\circ) = \boxed{\sin 70^(\circ)} \approx \boxed{0.9397}`

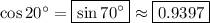
Note: if the computer doesn't like the answer of "sin 70" then try "sin(70)" and see how that works out. It's also possible that the teacher wants the single numeric value of "70" in the first box rather than the "sine" part to go along with it. Though that would be strange and incorrect since cos(20) = 70 is a false statement.