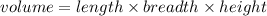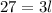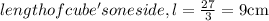Question

Bill has a perfect cube of an unknown substance. The density of the substance is 3.0 g/cm. He

found the mass of the cube to be 81 9. What is the length of one side of the cube?
Find the volume first:
volume from calculation = ___cm x__cm x__cm (vol. of a cube)

Answer 1

The volume of the cube of unknown substance is
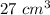`27\ cm^3`. The length of one side of the cube is 9 cm

Step-by-step explanation:

As per the formula of mass and density, Mass / Density equals to volume. Therefore as the mass of the cube was given to be 81 g and the density of the cube is known to be 3.0 g/
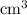`\mathrm{cm}^(3)`,, to help Bill find out the volume. It is the ratio of mass and density and can be calculated as

`\text {volume}=\frac{\text {mass}}{\text {density}}=\frac{81 g}{3 g / \mathrm{cm}^(3)}=27 \mathrm{cm}^(3)`

As it is a cube, the volume is product of length, breadth, and height. Here, all sides are same as it is the cube.

`volume= length * breadth * height`

`27=3 l`

`length of cube's one side, l=(27)/(3)=9 \mathrm{cm}`