Question

When a certain string is clamped at both ends, the lowest four resonant frequencies are 50, 100, 150, and 200 Hz. When the string is also clamped at its midpoint, the lowest four resonant frequencies are __________.

Answer 1

Step-by-step explanation:

Given

Lowest four resonance frequencies are given with magnitude

50,100,150 and 200 Hz

The frequency of vibrating string is given by

`f=(n)/(2L)\sqrt{(T)/(\mu )}`

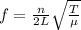
where n=1,2,3 or ...n

L=Length of string

T=Tension

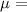`\mu =`Mass per unit length

When string is clamped at mid-point

Effecting length becomes
`L'=0.5 L`

Thus new Frequency becomes

`f' =(n)/(L)\sqrt{(T)/(\mu )}`

i.e. New frequency is double of old

so new lowest four resonant frequencies are 100,200,300 and 400 Hz