Answer:
Therefore Anjeli is correct and
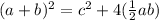 is TRUE.
is TRUE.
Explanation:
Given:
Label on triangle as ΔABC right angle at C such that
AB = c .....(Hypotenuse)
AC = b ....(Longer leg)
BC = a ....(Shorter leg)
To Prove:
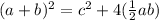
Proof:
We know, in Right angle triangle ABC by Pythagoras theorem we get,
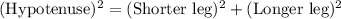
Substituting the values we get
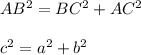 ...............( 1 )
...............( 1 )
Now the Left hand side of what Anjeli wrote is
Left hand side = (a+b)²
Using identity (A+B)² = A²+ B² + 2AB we get
Left hand side = a²+ b² + 2ab
From ( 1 ) we have

Substituting we get
Left hand side = c² + 2ab ...........................( 2 )
Now,
Right hand side =
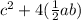
Dividing 4 by 2 we get 2, hence
Right hand side =
 .........................( 3 )
.........................( 3 )
Therefore,
Left hand side = Right hand side From ( 2 ) and ( 3 )
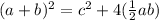 ..True
..True
Therefore Anjeli is correct and
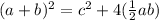 is TRUE.
is TRUE.