Answer:
1.
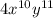
2.
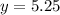
Explanation:
Question 1.
Given:
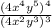
We need to Simplify given expression we get;
Solution:
Now we know that;
By Using Law of Indices which states;
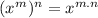
So we get:
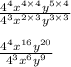
Now Again By Law of Indices we get;
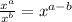
So we get:
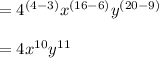
Hence Simplified expression is
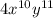 .
.
Question 2.
Given:
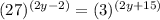
We need to solve for 'y'.
Solution:
To find 'y' we need to first make the base same.
Now we know that;
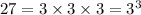
So we can say that:
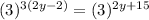
Now Applying Distributive property we get;
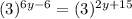
Now we can say that;
When an expression has equal bases then their exponent are said to equal too.
from above we get;

Combing like terms we get;
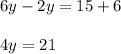
Dividing both side by 2 we get;
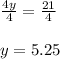
Hence The Value of y is 5.25.