Answer: The speed of the river's current is
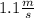
Explanation:
Let's begin by explaining that Speed is a relation between an object's traveled distance in a given time :
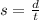
So, in this situation the man traveled a distance
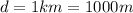 for a time
for a time
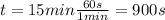 before realizing he lost his hat.
before realizing he lost his hat.
Keeping this information in mind we can calculate the speed of the river's current, since we already have a reference point: the bridge where the hat fell.

Then:
 This is the speed of the river's current
This is the speed of the river's current