Question

One of the roots of the quadratic equation x2−5mx+6m2=0 is 36. Find the greatest possible value of the second root. Help needed as soon as possible, Thank You. 50PTS!!

Answer 1

The greatest possible value of the second root is 54

Explanation:

Given quadratic equation is
`x^2-5mx+6m^2=0`

Now factorise the given quadratic equation :

`x^2-3mx-2mx+6m^2=0`

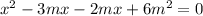
x(x-3m)-2m(x-3m)=0

(x-2m)(x-3m) = 0

x-2m=0 or x-3m=0

∴ x = 2m or x = 3m .

Therefore 2m and 3m are the roots of the given quadratic equation.

Given one of the roots of the quadratic equation is 36

Let 2m=36

`m=(36)/(2)`

`=18`

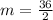
Therefore m=18

Substitute m=12 in 2m we have 2(12)=24

Now let 3m=36

`m=(36)/(3)`

`=12`

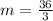
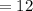
Therefore m=12

Substitute m=18 in 3m we have 3(18)=54

Therefore the greatest possible value of the second root is 54