Answer:
4m
Explanation:
- Recall the formula for area of a circle for each calculation
- Calculate the area of the large field (r = 5m)
- Calculate the area of the small field (r = 3m)
- Find the difference in area
- Find the radius of the "difference" field using difference in area
The formula for area of a circle is A = πr².
"A" means area.
π is pi. I will use the π button, but some teachers ask to use 3.14.
"r" means radius.
Area of large field:
Substitute "r" for 5m in the formula.
A = πr²
A = π(5m)²
A = π25m²
A = 78.5398163397 m²
Area of small field:
Substitute "r" for 3m in the formula.
A = πr²
A = π(3m)²
A = π9m²
A = 28.2743338823 m²
Difference in areas:
Subtract the area of small field from the area of large field.
(78.5398163397 m²) - (28.2743338823 m²)
= 50.2654825 m²
Radius of "difference" field:
Since we are looking for radius, not area, rearrange the formula to isolate "r".
A = πr²
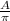 =
=
 Divide both sides by π
Divide both sides by π
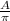 = r² π cancels out on the right side
= r² π cancels out on the right side
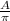 = √r² Square root both sides
= √r² Square root both sides
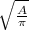 = r ² and √ cancel out, leaving "r" isolated
= r ² and √ cancel out, leaving "r" isolated
r =
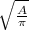 Variable on the left for standard formatting
Variable on the left for standard formatting
Substitute "A" for the difference in area
r =
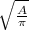
r =
 Divide by pi first
Divide by pi first
r =
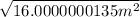 Find the square root
Find the square root
r ≈
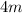
The radius of the field that has the area of the difference in the two fields is 4m.