1 Answer

Manuel Alvarez · Answer 1 · 2021-08-13T18:10:26+0000

The average rate of change is 2

Solution:

Given that we have to find the rate of change

The average rate of change is given as:

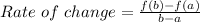
$Rate\ of\ change = (f(b)-f(a))/(b-a)$

Given function is:

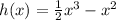
h(x) = (1)/(2)x^3 -x^2

Given interval is:

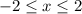
$-2\leq x\leq 2$

Therefore,

a = -2 and b = 2

Thus formula becomes:

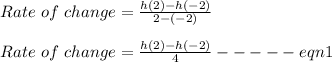
$Rate\ of\ change = (h(2)-h(-2))/(2-(-2))\\\\Rate\ of\ change = (h(2)-h(-2))/(4) ----- eqn 1$

Find h(2)

Substitute x = 2 in given function

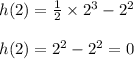
$h(2) = (1)/(2) * 2^3 - 2^2\\\\h(2) = 2^2-2^2 = 0$

Find h(-2)

Substitute x = -2 in given function

$h(-2) = (1)/(2) * (-2)^3 -(-2)^2\\\\h(-2) = -4 -4 = -8$

Substitute the values in eqn 1

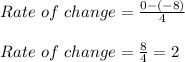
$Rate\ of\ change = (0-(-8))/(4)\\\\Rate\ of\ change =(8)/(4) = 2$

Thus average rate of change is 2

Please log in or register to add a comment.