Answer:
c(1)=-0.1
c(n)=c(n-1)*5
Explanation:
The recursive definition of a geometric sequence is given by:

where r is the common ratio.
The given given geometric sequence is −0.1 ,−0.5 ,−2.5 ,−12.5
c(1) is the first term.
This implies that:
c(1)=-0.1
To find the common ratio, we divide the subsequent terms of any two consecutive term by the previous term
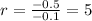
The recursive formula is
c(1)=-0.1
c(n)=c(n-1)*5