Question

g Calculate the activation energy (considering the Arrhenius expression) for a process that proceeds at 9x10-14 mol/sec at a temperature of 600K and at a rate of 6x10-11 mol/sec at 850K.

Answer 1

Answer: Activation energy

`E_(a)=110.243 kJ/mol`

Step-by-step explanation:

Arrhenius expression activation energy is given by

`k=Ae^{(-E_(a))/(RT)`

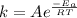
where
`k` is the rate constant,
`A` is the pre-exponential factor

`R`=The gas constant is 8.314 J / mol·K and
`T` is temperature

By substituting the values in the above equation we get two equations

`k_(1)=Ae^{(-E_(a))/(RT_(1))}\\9* 10^(-14)=Ae^{(-E_(a))/(8.314* 600)}...................(1)\\k_(2)=Ae^{(-E_(a))/(RT_(2))}\\6* 10^(-11)=Ae^{(-E_(a))/(8.314* 850)}...................(2)`

by solving 1 and 2, we get