Question

In 2017, the company SpaceX became the first private company to send supplies to the International Space Station with a reusable rocket. At launch, the total mass of the rocket was 552 Mg, and the thrust force exerted by the rocket engines was 7.61 MN. What was the magnitude of the rocket's initial acceleration

Answer 1

Approximately
`3.98\; \rm m \cdot s^(-2)`.

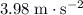Assumption: air resistance on the rocket is negligible. Take
`g = \rm 9.81\; m \cdot s^(-2)`.

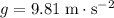Step-by-step explanation:

By Newton's Second Law of Motion, the acceleration of the rocket is proportional to the net force on it.

`\displaystyle \text{Acceleration} = \frac{\text{Net Force}}{\text{Mass}}`.

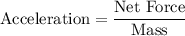Note that in this case, the uppercase letter
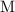`\rm M` in the units stands for "mega-", which is the same as
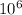`10^6` times the unit that follows. For example,
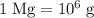`\rm 1\; Mg = 10^6\; g`, while
`\rm 1\; MN = 10^6\; N`.

Convert the mass of the rocket and the thrust of its engines to SI standard units:

• The standard unit for mass is kilograms:
  `\displaystyle m = \rm 552\; Mg = 552 * 10^6\; g * (1\; \rm kg)/(10^3\; g) = 552 * 10^3 \; kg`.
• The standard for forces (including thrust) is Newtons:
  `\text{Thrust} = \rm 7.61 \; MN = 7.61 * 10^6\; N`.

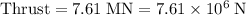At launch, the velocity of the rocket would be pretty low. Hence, compared to thrust and weight, the air resistance on the rocket would be pretty negligible. The two main forces that contribute to the net force of the rocket would be:

• Thrust (which is supposed to go upwards), and
• Weight (downwards due to gravity.)

The thrust on the rocket is already known to be
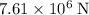`\rm 7.61 * 10^6\; N`. Since the rocket is quite close to the ground, the gravitational acceleration on it should be approximately
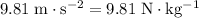`9.81\; \rm m \cdot s^(-2) = 9.81 \; N \cdot kg^(-1)`. Hence, the weight on the rocket would be approximately
`9.81\; \rm N \cdot kg^(-1) * 552 * 10^3\; kg = 5.41412* 10^6\; N`.

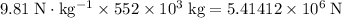The magnitude of the net force on the rocket would be

`\begin{aligned}&\text{Thrust} - \text{Weight} \\ &= 7.61 * 10^6\; \rm N - 5.41412* 10^6\; N \\ &\approx 2.19 * 10^6\; \rm N\end{aligned}`.

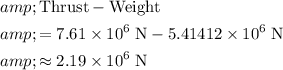Apply the formula
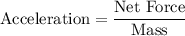`\displaystyle \text{Acceleration} = \frac{\text{Net Force}}{\text{Mass}}` to find the net force on the rocket. To make sure that the output (acceleration) is in SI units (meters-per-second,) make sure that the inputs (net force and mass) are also in SI units (Newtons for net force and kilograms for mass.)

`\begin{aligned}\displaystyle &\text{Acceleration} \\ &= \frac{\text{Net Force}}{\text{Mass}} \\ &= (2.19 * 10^6\; \rm N)/(552 * 10^3\; \rm kg) \\ &\approx \rm 3.98\; \rm m \cdot s^(-2)\end{aligned}`.